手把手构建LSTM的向前传播(Building a LSTM step by step)

 

本篇是在之前两篇基础上接着写的:

吴恩达deepLearning.ai循环神经网络RNN学习笔记(理论篇)

从头构建循环神经网络RNN的向前传播(rnn in pure python)

也可以不看，如果以下有看不懂的，再回过头来看上面两篇也行。

​

前言

目录

• 阀门和状态描述
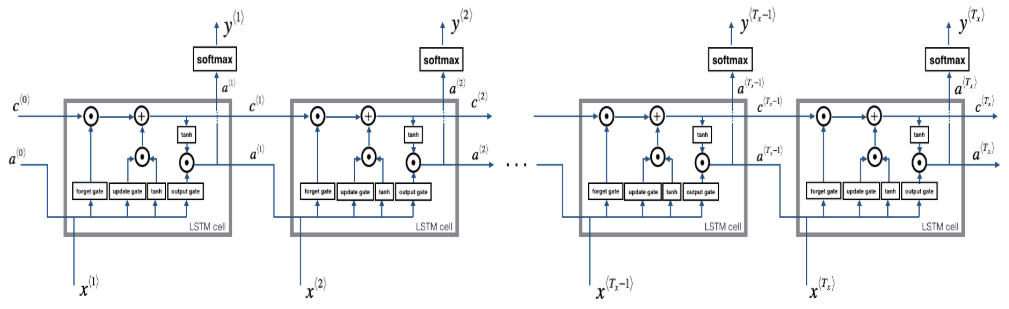
• LSTM cell
• LSTM整个过程

需要理解:

• 遗忘门，更新门，输出门的作用是什么，它们是怎么发挥作用的。
• 单元状态 cell state 是如何来选择性保留信息。

下面这张图将示意LSTM的操作。

LSTM单元，它在每一个时间步长跟踪更新“单元状态”或者是记忆变量。

 

同之前讲的RNN例子一样，我们将以一个时间步长的LSTM单元执行开始，接着你就可以用for循环处理Tx个时间步长。

 

​阀门和状态概述

遗忘门

 

概念:

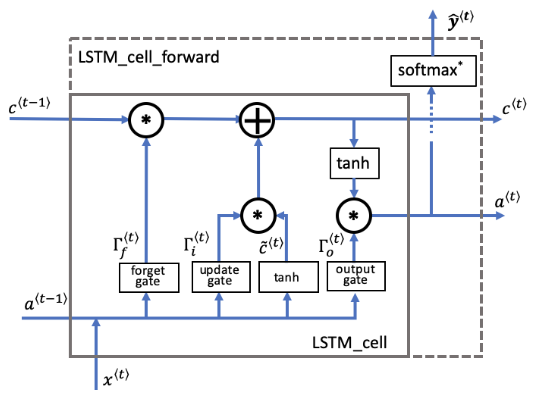
• 假设我们正在阅读一段文本中的单词，并计划使用LSTM跟踪语法结构，例如判断主体是单数（“ puppy”）还是复数（“ puppies”）。
• 如果主体更改其状态（从单数词更改为复数词），那么先前的记忆状态将过时，因此我们“忘记”过时的状态。
• “遗忘门”是一个张量，它包含介于0和1之间的值。
• 如果遗忘门中的一个单元的值接近于0，则LSTM将“忘记”之前单元状态相应单位的存储值。
• 如果遗忘门中的一个单元的值接近于1，则LSTM将记住大部分相应的值。

公式:

 

公式的解释:

• 包含控制遗忘门行为的权重。
• 之前时间步长的隐藏状态和当前时间步长的输入连接在一起乘以。
• sigmoid函数让每个门的张量值在0到1之间。
• 遗忘门和之前的单元状态有相同的shape。
• 这就意味着它们可以按照元素相乘。
• 将张量和相乘相当于在之前的单元状态应用一层蒙版。
• 如果中的单个值是0或者接近于0，那么乘积就接近0.
• 这就是使得存储在对应单位的值在下一个时间步长不会被记住。
• 同样，如果中的1个值接近于1，那么乘积就接近之前单元状态的原始值。
• LSTM就会在下一个时间步长中保留对应单位的值。

在代码中的变量名:

• Wf: 遗忘门的权重
• Wb: 遗忘门的偏差
• ft: 遗忘门

候选值

 

概念:

• 候选值是包含当前时间步长信息的张量，它可能会存储在当前单元状态中。
• 传递候选值的哪些部分取决于更新门。
• 候选值是一个张量，它的范围从-1到1。
• 代字号“〜”用于将候选值与单元状态区分开。

公式:

 

公式的解释:

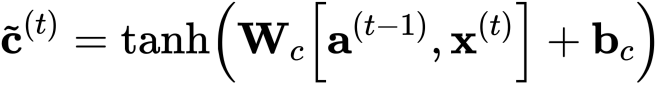
• 'tanh'函数产生的值介于-1和+1之间。

在代码中的变量名:

• cct: 候选值

---

更新门

 

概念:

• 我们使用更新门来确定候选的哪些部分要添加到单元状态中。
• 更新门是包含0到1之间值的张量。
• 当更新门中的单位接近于0时，它将阻止候选值中的相应值传递到。
• 当更新门中的单位接近1时，它允许将候选的值传递到。
• 注意，我们使用下标“i”而不是“u”来遵循文献中使用的约定。

 

公式:

 

公式的解释:

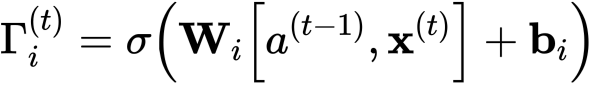
• 类似于遗忘门（此处为），用sigmoid函数乘后值就落在了0到1之间。
• 将更新门与候选元素逐元素相乘，并将此乘积()用于确定单元状态。

在代码中的变量名:

在代码中，我们将使用学术文献中的变量名。这些变量不使用“ u”表示“更新”。

• wi是更新门的权重
• bi是更新门的偏差
• it是更新门

---

 

单元状态

概念:

• 单元状态是传递到未来时间步长的“记忆/内存(memory)”。
• 新单元状态是先前单元状态和候选值的组合。

公式:

 

公式的解释:

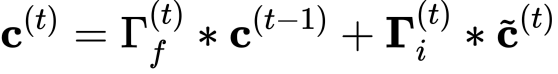
• 之前的单元状态通过遗忘门调整(加权)。
• 候选值通过更新门调整(加权)。

在代码中的变量名:

• c: 单元状态，包含所有的时间步长，c的shape是(na, m, T)
• c_next: 下一个时间步长的单元状态，的shape (na, m)
• c_prev: 之前的单元状态，的shape (na, m)

---

输出门

概念:

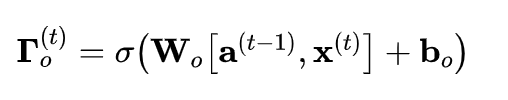
• 输出门决定时间步长要输出的预测值。
• 输出门与其他门一样，它包含从0到1的值。

公式:

公式的解释:

• 输出门由之前的隐藏状态和当前的输入 决定。
• sigmoid函数让值的范围在0到1之间。

在代码中的变量名:

• wo: 输出门的权重
• bo: 输出门的偏差
• ot: 输出门

---

隐藏状态

 

概念:

• 隐藏状态将传递到LSTM单元的下一个时间步长。
• 它用于确定下一个时间步长的三个门()。
• 隐藏状态也用于预测。

公式:

公式的解释:

• 隐藏状态由单元状态结合输出门确定。
• 单元状态通过“ tanh”函数把值缩放到-1和+1之间。
• 输出门的作用就像一个“掩码mask”，它既可以保留的值，也可以使这些值不包含在隐藏状态中。

在代码中的变量名:

• a: 隐藏状态，包含时间步长，shape (na, m, Tx)
• a_prev: 前一步的隐藏状态，的shape (na, m)
• a_next: 下一步的隐藏状态，的shape (na, m)

---

预测值

概念:

• 此用例的预测是分类，所以我们用softmax。

公式:

 

 

在代码中的变量名:

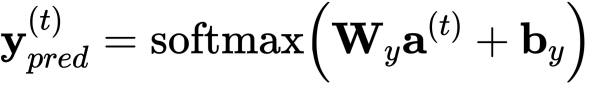
• y_pred: 预测，包含所有的时间步长，的shape (ny, m, Tx)，注意，本例中Tx=Ty。
• yt_pred: 当前时间步长t的预测值，shape是(ny, m)

LSTM cell‍

一共三个步骤:

1. 连接隐藏状态和输入成一个单独的矩阵

2. 依次计算上面那6个公式

3. 计算预测值

 

def lstm_cell_forward(xt, a_prev, c_prev, parameters):
    """
    Implement a single forward step of the LSTM-cell as described in Figure (4)
​
    Arguments:
    xt -- your input data at timestep "t", numpy array of shape (n_x, m).
    a_prev -- Hidden state at timestep "t-1", numpy array of shape (n_a, m)
    c_prev -- Memory state at timestep "t-1", numpy array of shape (n_a, m)
    parameters -- python dictionary containing:
                        Wf -- Weight matrix of the forget gate, numpy array of shape (n_a, n_a + n_x)
                        bf -- Bias of the forget gate, numpy array of shape (n_a, 1)
                        Wi -- Weight matrix of the update gate, numpy array of shape (n_a, n_a + n_x)
​                        bi -- Bias of the update gate, numpy array of shape (n_a, 1)
                        Wc -- Weight matrix of the first "tanh", numpy array of shape (n_a, n_a + n_x)
                        bc --  Bias of the first "tanh", numpy array of shape (n_a, 1)
                        Wo -- Weight matrix of the output gate, numpy array of shape (n_a, n_a + n_x)
                        bo --  Bias of the output gate, numpy array of shape (n_a, 1)
                        Wy -- Weight matrix relating the hidden-state to the output, numpy array of shape (n_y, n_a)
                        by -- Bias relating the hidden-state to the output, numpy array of shape (n_y, 1)
​
    Returns:
    a_next -- next hidden state, of shape (n_a, m)
    c_next -- next memory state, of shape (n_a, m)
    yt_pred -- prediction at timestep "t", numpy array of shape (n_y, m)
    cache -- tuple of values needed for the backward pass, contains (a_next, c_next, a_prev, c_prev, xt, parameters)
​
    Note: ft/it/ot stand for the forget/update/output gates, cct stands for the candidate value (c tilde),
          c stands for the cell state (memory)
    """
    # 从 "parameters" 中取出参数。
    Wf = parameters["Wf"] # 遗忘门权重
    bf = parameters["bf"]
    Wi = parameters["Wi"] # 更新门权重 (注意变量名下标是i不是u哦)
    bi = parameters["bi"] # (notice the variable name)
    Wc = parameters["Wc"] # 候选值权重
    bc = parameters["bc"]
    Wo = parameters["Wo"] # 输出门权重
    bo = parameters["bo"]
    Wy = parameters["Wy"] # 预测值权重
    by = parameters["by"]
    # 连接 a_prev 和 xt
    concat = np.concatenate((a_prev, xt), axis=0)
    # 等价于下面代码
    # 从 xt 和 Wy 中取出维度
    # n_x, m = xt.shape
    # n_y, n_a = Wy.shape
    # concat = np.zeros((n_a + n_x, m))
    # concat[: n_a, :] = a_prev
    # concat[n_a :, :] = xt
    # 计算 ft (遗忘门), it (更新门)的值
    # cct (候选值), c_next (单元状态),
    # ot (输出门), a_next (隐藏单元)
    ft = sigmoid(np.dot(Wf, concat) + bf)        # 遗忘门
    it = sigmoid(np.dot(Wi, concat) + bi)        # 更新门
    cct = np.tanh(np.dot(Wc, concat) + bc)       # 候选值
    c_next = ft * c_prev + it * cct    # 单元状态
    ot = sigmoid(np.dot(Wo, concat) + bo)        # 输出门
    a_next = ot * np.tanh(c_next)    # 隐藏状态
    # 计算LSTM的预测值
    yt_pred = softmax(np.dot(Wy, a_next) + by)
    # 用于反向传播的缓存
    cache = (a_next, c_next, a_prev, c_prev, ft, it, cct, ot, xt, parameters)
​
    return a_next, c_next, yt_pred, cache

 

 

LSTM向前传播

 

我们已经实现了一个时间步长的LSTM，现在我们可以用for循环对它进行迭代，处理一系列的Tx输入。

LSTM的多个时间步长

 

指导:

• 从变量x 和 parameters中获得 的维度。
• 初始化三维张量 , 和 .
• : 隐藏状态, shape
• : 单元状态, shape
• : 预测, shape (注意在这个例子里 ).
• 注意 将一个变量设置来和另一个变量相等是"按引用复制". 换句话说，就是不用使用c = a, 否则这两个变量指的是同一个变量，更改任何其中一个变量另一个变量的值都会跟着变。
• 初始化二维张量
• 储存了t时间步长的隐藏状态，它的变量名是a_next。
• , 时间步长0时候的初始隐藏状态，调用该函数时候传入的值，它的变量名是a0。
• 和 代表单个时间步长，所以他们的shape都是
• 通过传入函数的初始化隐藏状态来初始化 。
• 用0来初始化 。
• 变量名是 c_next.
• 表示单个时间步长, 所以它的shape是
• 注意: create c_next as its own variable with its own location in memory. 不要将它通过3维张量的切片来初始化，换句话说， 不要 c_next = c[:,:,0].
• 对每个时间步长，做以下事情:
• 从3维的张量 中, 获取在时间步长t处的2维切片 。
• 调用你之前定义的 lstm_cell_forward 函数，获得隐藏状态，单元状态，预测值。
• 存储隐藏状态，单元状态，预测值到3维张量中。
• 把缓存加入到缓存列表。

def lstm_forward(x, a0, parameters):
    """
    Implement the forward propagation of the recurrent neural network using an LSTM-cell described in Figure (4).
​
    Arguments:
    x -- Input data for every time-step, of shape (n_x, m, T_x).
    a0 -- Initial hidden state, of shape (n_a, m)
    parameters -- python dictionary containing:
                        Wf -- Weight matrix of the forget gate, numpy array of shape (n_a, n_a + n_x)
                        bf -- Bias of the forget gate, numpy array of shape (n_a, 1)
                        Wi -- Weight matrix of the update gate, numpy array of shape (n_a, n_a + n_x)
                        bi -- Bias of the update gate, numpy array of shape (n_a, 1)
                        Wc -- Weight matrix of the first "tanh", numpy array of shape (n_a, n_a + n_x)
                        bc -- Bias of the first "tanh", numpy array of shape (n_a, 1)
                        Wo -- Weight matrix of the output gate, numpy array of shape (n_a, n_a + n_x)
                        bo -- Bias of the output gate, numpy array of shape (n_a, 1)
                        Wy -- Weight matrix relating the hidden-state to the output, numpy array of shape (n_y, n_a)
                        by -- Bias relating the hidden-state to the output, numpy array of shape (n_y, 1)
​
    Returns:
    a -- Hidden states for every time-step, numpy array of shape (n_a, m, T_x)
    y -- Predictions for every time-step, numpy array of shape (n_y, m, T_x)
    c -- The value of the cell state, numpy array of shape (n_a, m, T_x)
    caches -- tuple of values needed for the backward pass, contains (list of all the caches, x)
    """
​
    # 初始化 "caches", 用来存储每个时间步长的cache值的
    caches = []

    Wy = parameters['Wy']
    # 从 x 和 parameters['Wy'] 的shape中获取纬度值
    n_x, m, T_x = x.shape
    n_y, n_a = Wy.shape
​
    # 初始化 "a", "c" and "y"
    a = np.zeros((n_a, m, T_x))
    c = np.zeros((n_a, m, T_x))
    y = np.zeros((n_y, m, T_x))
​
    # 初始化 a_next and c_next
    a_next = a0
    c_next = np.zeros(a_next.shape)
​
    # loop over all time-steps
    for t in range(T_x):
        # 从3维张量x中获取t时间步长的2维张量xt
        xt = x[:, :, t]
        # 更新下一个时间步长的隐藏状态, 下一个单元状态, 计算预测值
        a_next, c_next, yt, cache = lstm_cell_forward(xt, a_next, c_next, parameters)
        # 把下一个时间步长长的隐藏状态保存起来
        a[:,:,t] = a_next
        # 把下一个时间步长长的单元状态保存起来
        c[:,:,t]  = c_next
        # 把预测值保存起来
        y[:,:,t] = yt
        # 保存缓存值
        caches.append(cache)

    # 用于向后传播
    caches = (caches, x)
​
    return a, y, c, caches

恭喜你！现在，你已经为LSTM实现了前向传播。使用深度学习框架时，实施前向传播足以构建出色性能的系统。