Leetcode 69. Sqrt(x)及其扩展（有/无精度、二分法、牛顿法）详解

Leetcode 69. Sqrt(x) Easy

https://leetcode.com/problems/sqrtx/

Implement int sqrt(int x).

Compute and return the square root of x, where x is guaranteed to be a non-negative integer.

Since the return type is an integer, the decimal digits are truncated and only the integer part of the result is returned.

Example 1:

Input: 4
Output: 2

Example 2:

Input: 8
Output: 2
Explanation: The square root of 8 is 2.82842..., and since
             the decimal part is truncated, 2 is returned.

分析：

leetcode上的这个不带精度要求，且输出一个整数即可（其实可以当成精度要求小于等于1）。

方法一：（二分法）

对于本题，最直观的方法就是二分法。使用二分法时，需要注意有三个指针，分别指向前中后(pre、medium、last，在书写、习惯、理解上，一般用left、mid、right进行表示，或者用start、mid、end)。此外，循环结束的条件也需要在书写程序之前想好，当然这也是本题的难点。

边界条件怎么确定呢？
假设left < right时循环，当left等于right时循环结束，并返回left或right，此时有left=right=mid。那么可以取一个整数进行实验：比如8。
第一次循环:
mid = (1 + 8)/2 = 4 （整数除法默认向下取整）
mid > 8/mid
right = mid - 1 = 3
left = 1
第二次循环：
mid = (1 + 3)/2 = 2
mid < 8/mid
left = mid + 1 = 3
right = 3
此时由于left == right 则跳出循环，返回的是3，但是这不应该是正确答案。如果在循环条件中加上等于，即left <= right,那么下一步还会有一次循环：
第三次循环：（新增的）
mid = 3
mid > 8/mid
right = mid - 1 = 2
left = 3
此时left > righ结束循环。写到这里，我们发现，如果在循环结束时，对于此例子，返回right是比较合适的。这是个例吗，还是都是这样?

比如x=10，那么mid的变化过程将是：5->2->3,此时10/3 == 3，直接返回了。再如x=11，那么mid变化过程将是：5->2->3,此时11/3 == 3，直接返回了。

再比如x=12，那么mid变化过程将是：6->3->4(此时left=4，right=5)->4(left=4,right=4)->此时right-1，变为left = 4， right = 3，返回right。
所以right必为返回值（在不存在mid == x / mid的条件下）

至于为什么，可以细想一下，我也没想明白，给出理论解释。直观感觉是开根号为向下取整，而返回right时，right < left，正好算是向下取整。

注意：之所以要写成除法的形式，是因为如果两个大数相乘，容易超内存。

int mySqrt(int x) {
    int left = ;  // left不能取0;因为如果x=1，那么（0+1）/2 = 0，导致mid等于0，做除法的时候会报错
    int right = x;
    while (left <= right) {
        // int mid = (left + right) / 2;  ——> 不要这么写，是因为如果right很大，left+right可能会超过整型最大值
        int mid = left + (right - left) / 2;
        if (mid == x / mid) {
            return mid;
        }
        else if (mid > x / mid) {
            right = mid - ;
        }
        else {  // mid < x / mid
            left = mid + ;
        }
    }
    return right;
}

方法二：（牛顿法）

下面介绍牛顿法/牛顿迭代法。使用牛顿法千万不要死记硬背公式，要明白推导过程。牛顿法是用来求方程的近似根。通过使用f(x)的泰勒级数的前几项来寻找f(x)=0的根。（思考，和xgboost中使用牛顿法有什么区别）

关于泰勒级数的介绍：（说的不错）

https://baike.baidu.com/item/%E6%B3%B0%E5%8B%92%E7%BA%A7%E6%95%B0

https://zh.wikipedia.org/wiki/%E6%B3%B0%E5%8B%92%E7%BA%A7%E6%95%B0

关于牛顿法/牛顿迭代法：

https://baike.baidu.com/item/%E7%89%9B%E9%A1%BF%E8%BF%AD%E4%BB%A3%E6%B3%95 （百科介绍的太好了）

https://www.zhihu.com/question/20690553

https://www.cnblogs.com/wangkundentisy/p/8118007.html

（介绍了牛顿迭代法的使用，主要对问题进行求根转化后，然后求切线与x轴交点，并进行迭代更新此处更适合用来求根/零点，和下面这个优化算法是不太一样的）

https://blog.csdn.net/google19890102/article/details/41087931

（作为优化算法的牛顿法，整体思想就是利用迭代点处的一阶导数(梯度)和二阶导数(Hessen矩阵)对目标函数进行二次函数近似，然后把二次模型的极小点作为新的迭代点，并不断重复这一过程，直至求得满足精度的近似极小值。这就和XGBoost对损失函数进行二阶泰勒展开是相同的原理，且用目标函数对f_t-1(x)求一阶导数，所以说利用了牛顿法；而且在XGBoost中，求得的f_t-1(x)或者说w_q(x)即是下一次更新的叶子节点的权重。结合着论文和这篇博客还是比较容易懂，值得反复看）

为什么优化和求根都叫牛顿法呢？明明在求根和零点问题上，就是作切线嘛，明明没有泰勒展开啊，看看百度百科的“回答”（让我豁然开朗）：

注意的关键词：非线性方程，泰勒级数，取线性部分，近似方程。

下面进行详细分析，推导出迭代关系式（用iPad手写推导过程），并给出代码：

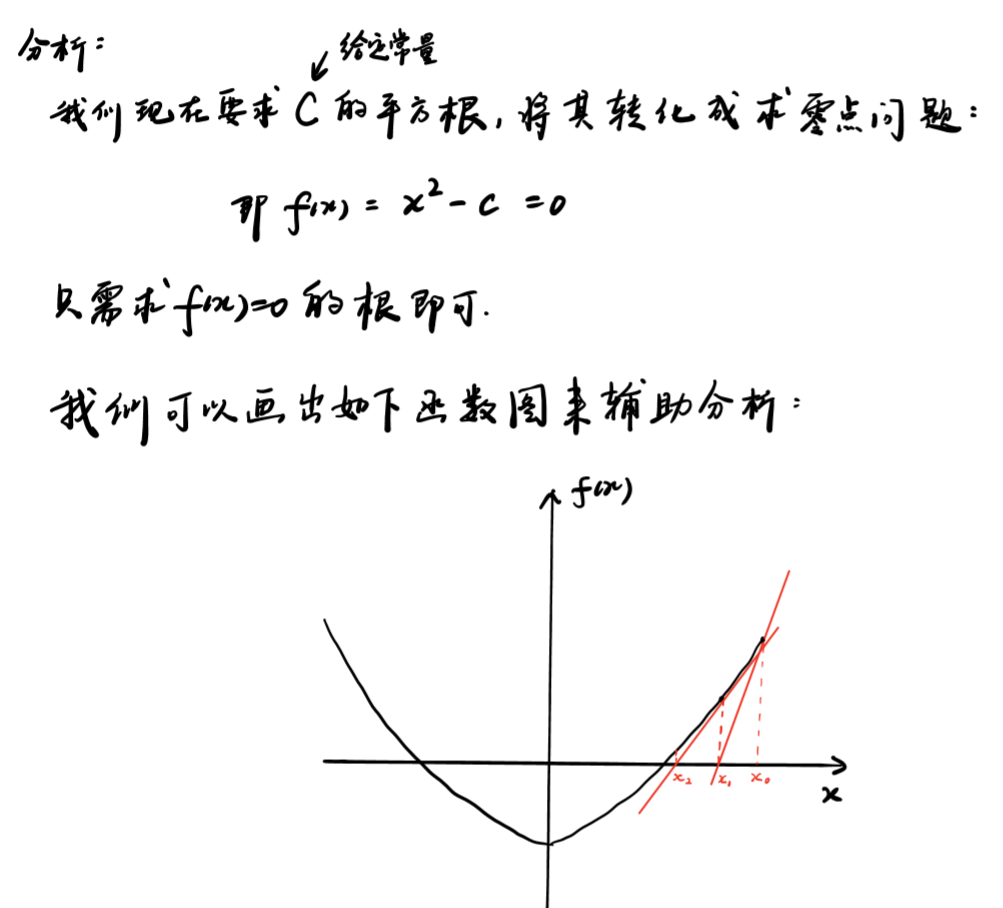
 总结：

由上分析我们可以发现，由牛顿法和切线法得来的递推公式是相同的，我们姑且可以认为切线法是牛顿法的几何表示。

此时，所谓的牛顿法对我已不再神秘，希望对你也一样如此！

int mySqrt(int x) {
    long long ans = x;
    while (ans * ans > x) {  // 其实，我很疑惑：如果ans很大的情况下，ans*ans不应该会报错吗
        ans = (ans + x / ans) / ; // 由公式化简得来
    }
    return ans;
}

至此，Leetcode上的这道 “Easy” 题目已经解决。但是往往在面试或实际问题中，要求最后得到的结果具备一定精度，即ans*ans - x < ε。此外，如果要求 ans值满足某一精度，我们就必须使用sqrt()求出其真实开根号值，然后作为判断条件。下面对一种进行代码书写，整体思路和不带精度相同，但是要注意需要把int型转为double型！

二分法：

double mySqrt(double x, double epsilon) {
    double left = 1.0;
    double right = x;
    double mid = left + (right - left) / ;
    while (fabs(mid * mid - x) > epsilon) {  // 默认mid * mid不会超过范围，否则这道题就麻烦了
        if (mid * mid > x) {
            right = mid;
        }
        else if (mid * mid < x) {
            left = mid;
        }
        else {
            return mid;
        }
        mid = left + (right - left) / ;
    }
    return mid;
}

牛顿法：

double mySqrt(double x, double epsilon) {
    double ans = x;
    while (fabs(ans * ans - x) > epsilon) {
        ans = (ans + x / ans) / ;
    }
    return ans;
}

注：

关于数学符号表示的知识，如：epsilon ：ε