AtCoder Beginner Contest 086 D - Checker

Time limit : 2sec / Memory limit : 256MB

Score : 500 points

Problem Statement

AtCoDeer is thinking of painting an infinite two-dimensional grid in a checked pattern of side K. Here, a checked pattern of side K is a pattern where each square is painted black or white so that each connected component of each color is a K × K square. Below is an example of a checked pattern of side 3:

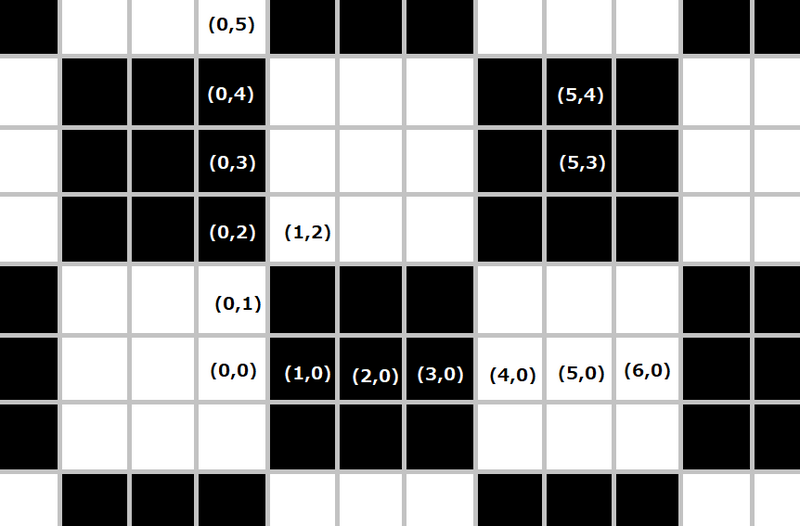
AtCoDeer has N desires. The i-th desire is represented by x_i, y_i and c_i. If c_i is B, it means that he wants to paint the square (x_i,y_i) black; if c_i is W, he wants to paint the square (x_i,y_i) white. At most how many desires can he satisfy at the same time?

Constraints

• 1 ≤ N ≤ 10⁵
• 1 ≤ K ≤ 1000
• 0 ≤ x_i ≤ 10⁹
• 0 ≤ y_i ≤ 10⁹
• If i ≠ j, then (x_i,y_i) ≠ (x_j,y_j).
• c_i is B or W.
• N, K, x_i and y_i are integers.

---

Input

Input is given from Standard Input in the following format:

N K
x1 y1 c1
x2 y2 c2
:
xN yN cN

Output

Print the maximum number of desires that can be satisfied at the same time.

---

Sample Input 1

Copy

4 3
0 1 W
1 2 W
5 3 B
5 4 B

Sample Output 1

Copy

4

He can satisfy all his desires by painting as shown in the example above.

---

Sample Input 2

Copy

2 1000
0 0 B
0 1 W

Sample Output 2

Copy

2

---

Sample Input 3

Copy

6 2
1 2 B
2 1 W
2 2 B
1 0 B
0 6 W
4 5 W

Sample Output 3

Copy

4

这道题数据挺大的，首先是移动到2k*2k的框框里，不影响坐标（也就是横纵坐标都移动2k的整数倍,如果只移动k的整数倍不能保证颜色不变）。
最初先默认数黑色或者白色都可以，这里先默认数黑色，白色的通过变色移动（横或纵坐标+k）映射到黑色的位置，然后整个图里只剩下黑色的。
最后求出结果，只需要取sum 和 n - sum中大的那个就行了，因为默认是黑色，黑白可以互换。
然后是移动k宫格在不同位置，一共有k*k种情况，直接在2k*2k的框框里操作，需要计算二维前缀和，之前是一个一个点去判断超时了，想想也不能一味的暴力啊，肯定得用点妙招，然后移动x轴，计算一维前缀和时间勉强没超。
k宫格是从左下角开始移动就是从（0,0）开始，需要记录的是k宫格里黑色的个数，以及斜对角的矩形里黑色的个数，因为斜对角的颜色相同，横竖相邻的区域颜色相异。
计算二维前缀和需要用离散学的容斥定理，最后求单独区域需要前缀和相减，也要用到容斥定理。
代码：

#include <iostream>
#include <algorithm>
#include <cstdio>
#include <cstdlib>
#include <cstring>
#include <cmath>
#include <iomanip>

using namespace std;

int v[][];
int n,k;
int num(int x1,int y1,int x2,int y2){
    return v[x2][y2] - v[x2][y1] - v[x1][y2] + v[x1][y1];///前缀和 减去左边 下边和左下边的k宫格前缀和  容斥定理：左边和下边都包含了左下边 需要加上一个左下边
}
int main()
{
    int x,y,ans = 0,sum;
    char ch;
    scanf("%d%d",&n,&k);
    for(int i = ;i <= n;i ++){
        scanf("%d%d %c",&x,&y,&ch);
        x %=  * k;///把所有点都移动到 2k * 2k 的区域
        y %=  * k;
        x += k * (ch == 'W');///x可以变成y W 也可以变成 B 这里白色都变成黑色的 如果转换后的黑色是满足的那么原来的白色也一定是满足的
        v[x % ( * k) + ][y % ( * k) + ] ++;///求前缀和要求从（1,1）开始
    }
    for(int i = ;i <=  * k;i ++)///求前缀和
        for(int j = ;j <=  * k;j ++)
            v[i][j] += v[i - ][j] + v[i][j - ] - v[i - ][j - ];///加上左边下边和左下角的和  容斥定理：左边和下边的都包含了左下边的  要减去一个左下边的for(int i = ;i <= k;i++)///k * k个格子依次做为起点构成新的k宫格 也就是移动k宫格 看看有几个黑色点包含在内 （默认黑色 可互换）
        for(int j = ;j <= k;j++)
        {
            ///斜对角的k宫格是相同颜色 2k * 2k区域最多有五个这样的区域
            sum = num(,,i,j) + num(i,j,k + i,k + j) + num(k + i,k + j, * k, * k) + num(k + i,, * k,j) + num(,k + j,i, * k);
            ans = max(ans,max(sum,n - sum));///黑白色可以互换
        }
    printf("%d\n",ans);
}