Codeforces 626D Jerry's Protest(暴力枚举+概率)

D. Jerry's Protest

time limit per test：2 seconds

memory limit per test：256 megabytes

input：standard input

output：standard output

Andrew and Jerry are playing a game with Harry as the scorekeeper. The game consists of three rounds. In each round, Andrew and Jerry draw randomly without replacement from a jar containing n balls, each labeled with a distinct positive integer. Without looking, they hand their balls to Harry, who awards the point to the player with the larger number and returns the balls to the jar. The winner of the game is the one who wins at least two of the three rounds.

Andrew wins rounds 1 and 2 while Jerry wins round 3, so Andrew wins the game. However, Jerry is unhappy with this system, claiming that he will often lose the match despite having the higher overall total. What is the probability that the sum of the three balls Jerry drew is strictly higher than the sum of the three balls Andrew drew?

Input

The first line of input contains a single integer n (2 ≤ n ≤ 2000) — the number of balls in the jar.

The second line contains n integers a_i (1 ≤ a_i ≤ 5000) — the number written on the ith ball. It is guaranteed that no two balls have the same number.

Output

Print a single real value — the probability that Jerry has a higher total, given that Andrew wins the first two rounds and Jerry wins the third. Your answer will be considered correct if its absolute or relative error does not exceed 10^- 6.

Namely: let's assume that your answer is a, and the answer of the jury is b. The checker program will consider your answer correct, if .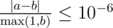

Examples

Input

2
1 2

Output

0.0000000000

Input

3
1 2 10

Output

0.0740740741

Note

In the first case, there are only two balls. In the first two rounds, Andrew must have drawn the 2 and Jerry must have drawn the 1, and vice versa in the final round. Thus, Andrew's sum is 5 and Jerry's sum is 4, so Jerry never has a higher total.

In the second case, each game could've had three outcomes — 10 - 2, 10 - 1, or 2 - 1. Jerry has a higher total if and only if Andrew won 2 - 1 in both of the first two rounds, and Jerry drew the 10 in the last round. This has probability .

题目链接：http://codeforces.com/contest/626/problem/D

题意：给定n个球以及每个球对应的分值a[]，现在A和B进行三局比赛，每局比赛两人随机抽取一个球进行比拼，分值高的获胜。现在A胜了两局，B不服输，因为他三局总分高于A。问发生的概率。

分析：首先分值最高为5000，可以考虑枚举分值求概率。假设B胜的那一局胜X分，A胜的两局胜Y分，我们可以考虑枚举X或者Y。以枚举X来说要求X > Y，关键在于求出B一局胜分X概率Pb[X] 以及 A两局胜分Y的概率Pa[Y]。

那么直接暴力就好了，暴力前sort一下。对于第i个球a[i]，胜分的球在j(1 <= j < i)，把所有胜分求出并统计cnt[]。这样对于一局比拼的胜分T，概率为cnt[T] / (n*(n-1)/2)。

求出一局的胜分，两局也就好求了。对于A而言，两局胜T分显然概率为cnt[a] / (n*(n-1)/2) * cnt[b] / (n*(n-1)/2) 其中(a + b == T)。A两局胜分T，可以O(a[max] * a[max])求出。

这题会爆int，所以。。。。。

下面给出AC代码：

 #include <bits/stdc++.h>
 using namespace std;
 typedef long long ll;
 const int N=;
 int n;
 double ans;
 ll cnt[N<<],a[N<<],b[N<<];
 inline int read()
 {
     int x=,f=;
     char ch=getchar();
     while(ch<''||ch>'')
     {
         if(ch=='-')
             f=-;
         ch=getchar();
     }
     while(ch>=''&&ch<='')
     {
         x=x*+ch-'';
         ch=getchar();
     }
     return x*f;
 }
 int main()
 {
     n=read();
     for(int i=;i<=n;i++)
         a[i]=read();
     sort(a+,a++n);
     for(int i=;i<=n;i++)
     {
         for(int j=n-;j>=;j--)
         {
             cnt[a[i]-a[j]]++;
         }
     }
     ll sum=(n-)*n/;
     for(int i=;i<=;i++)
     {
         for(int j=;j<=;j++)
         {
             b[i+j]+=1ll*cnt[i]*cnt[j];
         }
     }
     for(int i=;i<=;i++)
     {
         for(int j=i-;j>=;j--)
         {
             ans+=1.0*cnt[i]*b[j]/sum/sum/sum;
         }
     }
     printf("%.10lf\n",ans);
     return ;
 }