【POJ】2318 TOYS ——计算几何+二分

TOYS

Time Limit: 2000MS		Memory Limit: 65536K
Total Submissions: 10281		Accepted: 4924

Description

Calculate the number of toys that land in each bin of a partitioned toy box. 
Mom and dad have a problem - their child John never puts his toys away when he is finished playing with them. They gave John a rectangular box to put his toys in, but John is rebellious and obeys his parents by simply throwing his toys into the box. All the toys get mixed up, and it is impossible for John to find his favorite toys.

John's parents came up with the following idea. They put cardboard partitions into the box. Even if John keeps throwing his toys into the box, at least toys that get thrown into different bins stay separated. The following diagram shows a top view of an example toy box.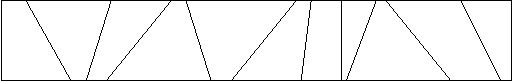 
 
For this problem, you are asked to determine how many toys fall into each partition as John throws them into the toy box.

Input

The input file contains one or more problems. The first line of a problem consists of six integers, n m x1 y1 x2 y2. The number of cardboard partitions is n (0 < n <= 5000) and the number of toys is m (0 < m <= 5000). The coordinates of the upper-left corner and the lower-right corner of the box are (x1,y1) and (x2,y2), respectively. The following n lines contain two integers per line, Ui Li, indicating that the ends of the i-th cardboard partition is at the coordinates (Ui,y1) and (Li,y2). You may assume that the cardboard partitions do not intersect each other and that they are specified in sorted order from left to right. The next m lines contain two integers per line, Xj Yj specifying where the j-th toy has landed in the box. The order of the toy locations is random. You may assume that no toy will land exactly on a cardboard partition or outside the boundary of the box. The input is terminated by a line consisting of a single 0.

Output

The output for each problem will be one line for each separate bin in the toy box. For each bin, print its bin number, followed by a colon and one space, followed by the number of toys thrown into that bin. Bins are numbered from 0 (the leftmost bin) to n (the rightmost bin). Separate the output of different problems by a single blank line.

Sample Input

5 6 0 10 60 0
3 1
4 3
6 8
10 10
15 30
1 5
2 1
2 8
5 5
40 10
7 9
4 10 0 10 100 0
20 20
40 40
60 60
80 80
 5 10
15 10
25 10
35 10
45 10
55 10
65 10
75 10
85 10
95 10
0

Sample Output

0: 2
1: 1
2: 1
3: 1
4: 0
5: 1

0: 2
1: 2
2: 2
3: 2
4: 2

Hint

As the example illustrates, toys that fall on the boundary of the box are "in" the box.

 

题意：给你一个矩形，并且用n根线段将矩形格成n+1个隔间，然后再给你m个点，让你统计每个隔间内点的个数。

 

题解：这题运用的是计算几何中点与线段的位置关系，用叉积的性质来判断点在线段的左边还是右边，再用二分搜索判断点所处的位置，并统计输出。

 

AC代码：

 

 #include <cstdio>
 #include <cstring>

 const int LEN = ;

 struct Point //点的结构体
 {
     int x;
     int y;
 };

 struct Line //线段的结构体
 {
     Point a;
     Point b;
 }line[LEN];

 int ans[LEN];

 int judge(Line tline, Point p3)  //运用叉积的性质判断点在线段的左边还是右边
 {
     Point t1, t2;
     t1.x = p3.x - tline.b.x;
     t1.y = p3.y - tline.b.y;
     t2.x = tline.a.x - tline.b.x;
     t2.y = tline.a.y - tline.b.y;
     return t1.x * t2.y - t1.y * t2.x;
 }

 int divide(int l, int r, Point toy) //二分查找
 {
     int mid = (l + r) / ;
     if (mid == l)
         return l;
     if (judge(line[mid], toy) < )
         return divide(l, mid, toy);
     else
         return divide(mid, r, toy);
 }

 int main()
 {
     int n, m, x1, y1, x2, y2;
     //freopen("in.txt", "r", stdin);
     while(scanf("%d", &n) != EOF && n){
         scanf("%d %d %d %d %d", &m, &x1, &y1, &x2, &y2);
         memset(ans, , sizeof(ans));
         for(int i = ; i <= n; i++){
             int up, low;
             scanf("%d %d", &up, &low);
             line[i].a.x = up;
             line[i].a.y = y1;
             line[i].b.x = low;
             line[i].b.y = y2;
         }
         n++;
         line[].a.x = line[].b.x = x1;
         line[].b.y = line[n].b.y = y2;
         line[].a.y = line[n].a.y = y1;
         line[n].a.x = line[n].b.x = x2;
         for(int i = ; i < m; i++){
             Point toy;
             scanf("%d %d", &toy.x, &toy.y);
             ans[divide(, n, toy)]++;
         }
         for(int i = ; i < n; i++){
             printf("%d: %d\n", i, ans[i]);
         }
         printf("\n");
     }
     return ;
 }