题解报告：poj 1195 Mobile phones（二维BIT裸题）

Description

Suppose that the fourth generation mobile phone base stations in the Tampere area operate as follows. The area is divided into squares. The squares form an S * S matrix with the rows and columns numbered from 0 to S-1. Each square contains a base station. The number of active mobile phones inside a square can change because a phone is moved from a square to another or a phone is switched on or off. At times, each base station reports the change in the number of active phones to the main base station along with the row and the column of the matrix. 
Write a program, which receives these reports and answers queries about the current total number of active mobile phones in any rectangle-shaped area. 

Input

The input is read from standard input as integers and the answers to the queries are written to standard output as integers. The input is encoded as follows. Each input comes on a separate line, and consists of one instruction integer and a number of parameter integers according to the following table. 

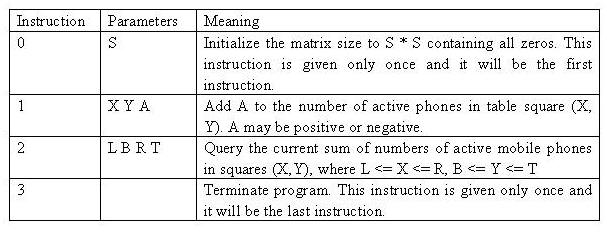
The values will always be in range, so there is no need to check them. In particular, if A is negative, it can be assumed that it will not reduce the square value below zero. The indexing starts at 0, e.g. for a table of size 4 * 4, we have 0 <= X <= 3 and 0 <= Y <= 3. 
Table size: 1 * 1 <= S * S <= 1024 * 1024 
Cell value V at any time: 0 <= V <= 32767 
Update amount: -32768 <= A <= 32767 
No of instructions in input: 3 <= U <= 60002 
Maximum number of phones in the whole table: M= 2^30 

Output

Your program should not answer anything to lines with an instruction other than 2. If the instruction is 2, then your program is expected to answer the query by writing the answer as a single line containing a single integer to standard output.

Sample Input

0 4
1 1 2 3
2 0 0 2 2
1 1 1 2
1 1 2 -1
2 1 1 2 3
3

Sample Output

3
4
解题思路：这是一道二维树状数组入门题---单点修改、单点（区间）查询，其思路和一维树状数组非常相似，多加了一个维度而已。下面我们来看看怎么实现这两个基本操作：
将一维数组A[]扩展到二维数组A[][]，二维树状数组C[][]来维护矩阵前缀和。
设原始二维数组A[][]={a11,a12,a13,a14,a15，
                     a21,a22,a23,a24,a25,
                     a31,a32,a33,a34,a35,
                     a41,a42,a43,a44,a45,
                     a51,a52,a53,a54,a55};
那么二维树状数组表示如下：
C[1][]=a1,C[1][]=a1+a1,C[1][]=a1,C[1][]=a1+a1+a1+a1,C[1][]=a15
这是数组A[][]第一行的一维树状数组；
C[][1]=a1+a1,C[][]=a1+a+a+a,C[][]=a+a,C[][]=a+a+a+a+a+a+a+a,C[][]=a+a5
这是数组A[][]第一行和第二行相加后得到的树状数组；
C[3][]=a3,C[3][]=a3+a3,C[3][]=a3,C[3][]=a3+a3+a3+a3,C[3][]=a35
这是数组A[][]第三行的一维树状数组；
C[][]=a+a+a+a,C[][]=a+a+a+a+a+a+a+a,C[][]=a+a+a+a...
这是数组A[][]前4行相加后得到的树状数组；
C[5][]=a5,C[5][]=a5+a5,C[5][]=a5,C[5][]=a5+a5+a5+a5,C[5][]=a55
这是数组A[][]第5行的一维树状数组。
仔细观察以上式子可以发现，二维树状数组C[x][y]的值其实是分别在x、y上的一维树状数组向下、向右（x上+lowbit(x)跳跃（>n停止），y上+lowbit(y)跳跃（>n停止））进行求和，这就是矩阵中坐标点值的单点修改。对于区间查询，同样分别在x、y上的一维树状数组从下往上，从右往左进行累加（y上-lowbit(y)跳跃（<=0停止），x上-lowbit(x)跳跃（<=0停止）），这样就得到了(1,1)到(x,y)矩阵中所有元素的和。
回到本题，题意为给出一些命令进行一些操作：
0 S 初始化一个全0的S*S矩阵，这个命令只会在第一次给出一次；
1 X Y A 给坐标点（X,Y）的值加上A；
2 L B R T 询问（L,B）到（R,T）构成的矩阵中所有元素的总和；
3 结束对矩阵的操作，程序终止。
典型的二维BIT，套一下模板即可，但需要注意一点：给出命令中的坐标都是默认从下标0开始的，为避免陷入死循环和计算错误，在更新和询问操作上统一对每一个坐标点（横、纵坐标）加1。
怎么统计坐标点（L,B）到（R,T）矩阵内所有值呢？给出下面的矩阵：
   1  2  3  4  5   
1  0  0  0  0  0 
2  0  0  0  0  0  
3     0  0  0  
4      0  0  0  
5    0  0  0  0 
从图上可得计算公式：[R,T]-[L-1,T]-[R,B-1]+[L-1,B-1](多减去了一个左上角的矩阵，还要把它加回来)，这样就得到了点（L,B）到（R,T）矩阵中所有元素的和。
AC代码：

 #include<cstdio>
 #include<string.h>
 const int maxn=;
 int op,s,x,y,a,l,b,r,t,C[maxn][maxn];
 void add(int x,int y,int val){//单点修改
     for(int i=x;i<=s;i+=i&-i)
         for(int j=y;j<=s;j+=j&-j)
             C[i][j]+=val;
 }
 int query(int x,int y){//前缀和查询
     int ans=;
     for(int i=x;i>;i-=i&-i)
         for(int j=y;j>;j-=j&-j)
             ans+=C[i][j];
     return ans;
 }
 int main(){
     while(~scanf("%d%d",&op,&s)){
         memset(C,,sizeof(C));
         while(~scanf("%d",&op)&&op!=){
             if(op==){
                 scanf("%d%d%d",&x,&y,&a);
                 x++,y++;add(x,y,a);//单点修改
             }
             else{
                 scanf("%d%d%d%d",&l,&b,&r,&t);l++,b++,r++,t++;
                 printf("%d\n",query(r,t)-query(l-,t)-query(r,b-)+query(l-,b-));//区间查询，求矩形中所有元素的和
             }
         }
     }
     return ;
 }