Power Network （最大流增广路算法模板题）

Time Limit: 2000MS		Memory Limit: 32768K
Total Submissions: 20754		Accepted: 10872

Description

A power network consists of nodes (power stations, consumers and dispatchers) connected by power transport lines. A node u may be supplied with an amount s(u) >= 0 of power, may produce an amount 0 <= p(u) <= p_max(u) of power, may consume an amount 0 <= c(u) <= min(s(u),c_max(u)) of power, and may deliver an amount d(u)=s(u)+p(u)-c(u) of power. The following restrictions apply: c(u)=0 for any power station, p(u)=0 for any consumer, and p(u)=c(u)=0 for any dispatcher. There is at most one power transport line (u,v) from a node u to a node v in the net; it transports an amount 0 <= l(u,v) <= l_max(u,v) of power delivered by u to v. Let Con=Σ_uc(u) be the power consumed in the net. The problem is to compute the maximum value of Con. 

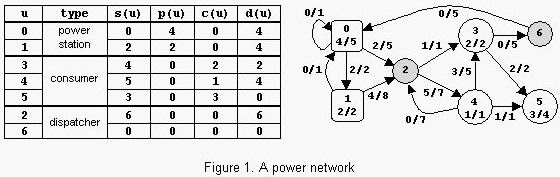
An example is in figure 1. The label x/y of power station u shows that p(u)=x and p_max(u)=y. The label x/y of consumer u shows that c(u)=x and c_max(u)=y. The label x/y of power transport line (u,v) shows that l(u,v)=x and l_max(u,v)=y. The power consumed is Con=6. Notice that there are other possible states of the network but the value of Con cannot exceed 6. 

Input

There are several data sets in the input. Each data set encodes a power network. It starts with four integers: 0 <= n <= 100 (nodes), 0 <= np <= n (power stations), 0 <= nc <= n (consumers), and 0 <= m <= n^2 (power transport lines). Follow m data triplets (u,v)z, where u and v are node identifiers (starting from 0) and 0 <= z <= 1000 is the value of l_max(u,v). Follow np doublets (u)z, where u is the identifier of a power station and 0 <= z <= 10000 is the value of p_max(u). The data set ends with nc doublets (u)z, where u is the identifier of a consumer and 0 <= z <= 10000 is the value of c_max(u). All input numbers are integers. Except the (u,v)z triplets and the (u)z doublets, which do not contain white spaces, white spaces can occur freely in input. Input data terminate with an end of file and are correct.

Output

For each data set from the input, the program prints on the standard output the maximum amount of power that can be consumed in the corresponding network. Each result has an integral value and is printed from the beginning of a separate line.

Sample Input

2 1 1 2 (0,1)20 (1,0)10 (0)15 (1)20
7 2 3 13 (0,0)1 (0,1)2 (0,2)5 (1,0)1 (1,2)8 (2,3)1 (2,4)7
         (3,5)2 (3,6)5 (4,2)7 (4,3)5 (4,5)1 (6,0)5
         (0)5 (1)2 (3)2 (4)1 (5)4

Sample Output

15
6

Hint

The sample input contains two data sets. The first data set encodes a network with 2 nodes, power station 0 with pmax(0)=15 and consumer 1 with cmax(1)=20, and 2 power transport lines with lmax(0,1)=20 and lmax(1,0)=10. The maximum value of Con is 15. The second data set encodes the network from figure 1.

 #include<stdio.h>
 #include<string.h>
 #include<queue>
 using namespace std;

 const int MAX = ;
 const int INF = 0x3f3f3f3f;
 int n,np,nc,m,mf,s,t;
 int cap[MAX][MAX],flow[MAX][MAX],a[MAX];
 int pre[MAX];
 char str[];
 queue <int> que;
 void maxflow()
 {
     memset(flow,,sizeof(flow));//初始化，所有的边的流量初始为0；
     mf = ;//记录最大流
     for(;;)
     {
         memset(a,,sizeof(a));//s到每个节点路径上的最小残量
         a[s] = INF;
         que.push(s);
         //bfs找增广路
         while(!que.empty())
         {
             int u = que.front();
             que.pop();
             for(int v = ; v <= n+; v++)
             {
                 if(!a[v] && cap[u][v] > flow[u][v])//找到新的节点v
                 {
                     pre[v] = u;//记录前驱并加入队列
                     que.push(v);
                     if(a[u] < cap[u][v]-flow[u][v])
                         a[v] = a[u];
                     else a[v] = cap[u][v]-flow[u][v];//s到v路径上的最小残量
                 }
             }
         }
         if(a[t] == ) break;//找不到最小残量，当前流已经是最大流；
         for(int u = t; u!= s;u = pre[u])//从汇点往回走
         {
             flow[pre[u]][u] += a[t];//更新正向流量
             flow[u][pre[u]] -= a[t];//更新反向流量
         }
         mf += a[t];//更新从s流出的总流量
     }
 }

 int main()
 {
     int u,v,z;
     while(~scanf("%d %d %d %d",&n,&np,&nc,&m))
     {
         memset(cap,,sizeof(cap));
         while(m--)
         {
            scanf("%s",str);
            sscanf(str,"(%d,%d)%d",&u,&v,&z);
            cap[u][v] = z;
         }

         while(np--)//有多个起点
         {
             scanf("%s",str);
             sscanf(str,"(%d)%d",&v,&z);
             cap[n][v] = z;//将多个起点连接到一个新的顶点作为起点；
         }

         while(nc--)//有多个终点
         {
             scanf("%s",str);
             sscanf(str,"(%d)%d",&u,&z);
             cap[u][n+] = z;//将多个终点连接到一个新的终点作为终点；
         }
         s = n;
         t = n+;
         maxflow();
         printf("%d\n",mf);
     }
     return ;
 }