AC日记——【模板】树链剖分 洛谷 P3384

题目描述

如题，已知一棵包含N个结点的树（连通且无环），每个节点上包含一个数值，需要支持以下操作：

操作1： 格式： 1 x y z 表示将树从x到y结点最短路径上所有节点的值都加上z

操作2： 格式： 2 x y 表示求树从x到y结点最短路径上所有节点的值之和

操作3： 格式： 3 x z 表示将以x为根节点的子树内所有节点值都加上z

操作4： 格式： 4 x 表示求以x为根节点的子树内所有节点值之和

输入输出格式

输入格式：

第一行包含4个正整数N、M、R、P，分别表示树的结点个数、操作个数、根节点序号和取模数（即所有的输出结果均对此取模）。

接下来一行包含N个非负整数，分别依次表示各个节点上初始的数值。

接下来N-1行每行包含两个整数x、y，表示点x和点y之间连有一条边（保证无环且连通）

接下来M行每行包含若干个正整数，每行表示一个操作，格式如下：

操作1： 1 x y z

操作2： 2 x y

操作3： 3 x z

操作4： 4 x

输出格式：

输出包含若干行，分别依次表示每个操作2或操作4所得的结果（对P取模）

输入输出样例

输入样例#1：

5 5 2 24
7 3 7 8 0
1 2
1 5
3 1
4 1
3 4 2
3 2 2
4 5
1 5 1 3
2 1 3

输出样例#1：

2
21

说明

时空限制：1s，128M

数据规模：

对于30%的数据：N<=10，M<=10

对于70%的数据：N<=1000，M<=1000

对于100%的数据：N<=100000，M<=100000

（其实，纯随机生成的树LCA+暴力是能过的，可是，你觉得可能是纯随机的么233）

样例说明：

树的结构如下：

各个操作如下：

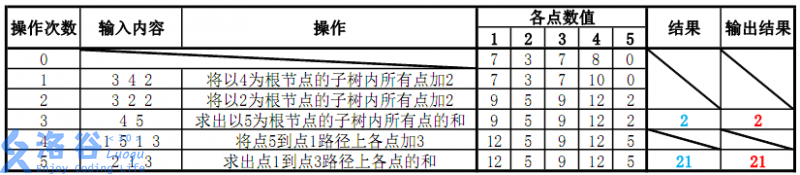
故输出应依次为2、21(重要的事情说三遍：记得取模)

思路：

　　裸树剖；

来，上代码：

#include <cstdio>
#include <cstring>
#include <iostream>
#include <algorithm>
 
#define maxn 100001
#define LL long long int
 
using namespace std;
 
struct EdgeType {
    LL to,next;
};
struct EdgeType edge[maxn<<];
 
struct TreeNodeType {
    LL l,r,dis,mid,flag;
};
struct TreeNodeType tree[maxn<<];
 
LL if_z,tree_num,tree_dis[maxn],deep[maxn],cnt;
LL f[maxn],n,m,s,p,dis[maxn],flag[maxn],Enum;
LL size[maxn],end[maxn],belong[maxn],head[maxn];
 
char Cget;
 
inline void read_int(LL &now)
{
    now=,if_z=,Cget=getchar();
    while(Cget>''||Cget<'')
    {
        if(Cget=='-') if_z=-;
        Cget=getchar();
    }
    while(Cget>=''&&Cget<='')
    {
        now=now*+Cget-'';
        Cget=getchar();
    }
    now*=if_z;
}
 
inline void edge_add(LL from,LL to)
{
    edge[++Enum].to=from,edge[Enum].next=head[to],head[to]=Enum;
    edge[++Enum].to=to,edge[Enum].next=head[from],head[from]=Enum;
}
 
inline void tree_up(LL now)
{
    tree[now].dis=tree[now<<].dis+tree[now<<|].dis;
}
 
void tree_build(LL now,LL l,LL r)
{
    tree[now].l=l,tree[now].r=r;
    if(l==r)
    {
        tree[now].dis=tree_dis[++tree_num];
        return ;
    }
    tree[now].mid=(tree[now].l+tree[now].r)>>;
    tree_build(now<<,l,tree[now].mid);
    tree_build(now<<|,tree[now].mid+,r);
    tree_up(now);
}
 
inline void tree_down(LL now)
{
    if(tree[now].l==tree[now].r) return ;
    tree[now<<].dis+=(tree[now<<].r-tree[now<<].l+)*tree[now].flag;
    tree[now<<].flag+=tree[now].flag;
    tree[now<<|].dis+=(tree[now<<|].r-tree[now<<|].l+)*tree[now].flag;
    tree[now<<|].flag+=tree[now].flag;
    tree[now].flag=;
}
 
void tree_change(LL now,LL l,LL r,LL x)
{
    if(tree[now].l==l&&tree[now].r==r)
    {
        tree[now].dis+=(r-l+)*x;
        tree[now].flag+=x;
        return ;
    }
    if(tree[now].flag) tree_down(now);
    if(l>tree[now].mid) tree_change(now<<|,l,r,x);
    else if(r<=tree[now].mid) tree_change(now<<,l,r,x);
    else
    {
        tree_change(now<<,l,tree[now].mid,x);
        tree_change(now<<|,tree[now].mid+,r,x);
    }
    tree_up(now);
}
 
LL tree_query(LL now,LL l,LL r)
{
    if(tree[now].l==l&&tree[now].r==r)
    {
        return tree[now].dis;
    }
    if(tree[now].flag) tree_down(now);
    tree_up(now);
    if(l>tree[now].mid) return tree_query(now<<|,l,r);
    else if(r<=tree[now].mid) return tree_query(now<<,l,r);
    else return tree_query(now<<,l,tree[now].mid)+tree_query(now<<|,tree[now].mid+,r);
}
 
void search(LL now,LL fa)
{
    LL pos=cnt++;
    deep[now]=deep[fa]+,f[now]=fa;
    for(LL i=head[now];i;i=edge[i].next)
    {
        if(edge[i].to==fa) continue;
        search(edge[i].to,now);
    }
    size[now]=cnt-pos;
}
 
void search_(LL now,LL chain)
{
    belong[now]=chain,flag[now]=++cnt;
    tree_dis[flag[now]]=dis[now];
    LL pos=;
    for(LL i=head[now];i;i=edge[i].next)
    {
        if(flag[edge[i].to]!=) continue;
        if(size[edge[i].to]>size[pos]) pos=edge[i].to;
    }
    if(pos!=) search_(pos,chain);
    for(LL i=head[now];i;i=edge[i].next)
    {
        if(flag[edge[i].to]!=) continue;
        search_(edge[i].to,edge[i].to);
    }
    end[now]=cnt;
}
 
inline void solve_change(LL x,LL y,LL z)
{
    while(belong[x]!=belong[y])
    {
        if(deep[belong[x]]<deep[belong[y]]) swap(x,y);
        tree_change(,flag[belong[x]],flag[x],z);
        x=f[belong[x]];
    }
    if(deep[x]<deep[y]) swap(x,y);
    tree_change(,flag[y],flag[x],z);
}
 
inline LL solve_query(LL x,LL y)
{
    LL ans=;
    while(belong[x]!=belong[y])
    {
        if(deep[belong[x]]<deep[belong[y]]) swap(x,y);
        ans=(ans+tree_query(,flag[belong[x]],flag[x]))%p;
        x=f[belong[x]];
    }
    if(deep[x]<deep[y]) swap(x,y);
    ans=(ans+tree_query(,flag[y],flag[x]))%p;
    return ans;
}
 
int main()
{
    read_int(n),read_int(m),read_int(s),read_int(p);
    for(LL i=;i<=n;i++) read_int(dis[i]);
    LL type,from,to,cur;
    for(LL i=;i<n;i++)
    {
        read_int(from),read_int(to);
        edge_add(from,to);
    }
    search(s,),cnt=,search_(s,s);
    cnt=,tree_build(,,n);
    for(LL i=;i<=m;i++)
    {
        read_int(type);
        if(type==)
        {
            read_int(from),read_int(to),read_int(cur);
            solve_change(from,to,cur);
        }
        if(type==)
        {
            read_int(from),read_int(to);
            printf("%d\n",solve_query(from,to)%p);
        }
        if(type==)
        {
            read_int(from),read_int(to);
            tree_change(,flag[from],end[from],to);
        }
        if(type==)
        {
            read_int(from);
            printf("%d\n",tree_query(,flag[from],end[from])%p);
        }
    }
    return ;
}